This semester i have seven subject which is the English, Trigonometry, Psychology, Computer Science Fundamentals, Computer Programming and Data Structure
English........ .............
Research Paper
A research paper is a piece of academic writing that requires a more abstract, critical, and thoughtful level of inquiry than you might be used to. But not to worry, you'll gradually pick up that mindset the more you envelop yourself in tutorial discussions and lectures at the college level, and of course, the more you write. Not just research papers but any paper, period.
Writing a research paper involves
(1) first familiarizing yourself with the works of "experts"--for example, on the page, in cyberspace, or in the flesh through personal interviews--to build upon what you know about a subject and then
(2) comparing their thoughts on the topic with your own.
Interview- is a conversation between two or more people (the interviewer and the interviewee) where questions are asked by the interviewer to obtain information from the interviewee.-is an action which helps interviewer who as an Employer, Candidate, Journalist, or an Ordinary person to make hypothesis about a person’s personality or a company’s organizational structure.
Step in conducting an interview
1. Set the feeling tone for the interview:
When you meet the person you will be interviewing so introduce yourself and thank the person for agreeing to do the interview. Explain the purpose of the interview and how you plan to use it. Try to make the interviewee as comfortable as possible. Having good sensitivity in the interview is very important. Be prepared to deal with painful moments with humanity and sensitivity and also be thoughtful and caring during the interview.
2. Set up Equipment:
Don’t make a big deal about the equipment. Get it set up and test it by asking the interviewee to state their name and address into the microphone. We suggest using clip on lavaliere mikes vs. stand up mikes. Be sure that the mike is turned on and the tape recorder volume is set properly. It is wise to bring a partner or friend along to keep track of the equipment while you concentrate on the interview. Common sense dictates that you bring a second person along to interview someone in his or her home.
3. Prepare the Person:
Say the following to the person you are interviewing “Please just share your story with us today. Share any memories you may have. The more you talk, the better. I’ll ask questions at the end.”
4. Start the Tape Recorders:
When you are ready to start the interview, make sure the tape recorders are turned on and the cassette tapes are recording. Use two recorders in case one fails. Start out the interview by saying, “I’m talking with ______ who is going to be sharing his/her experiences during (name of event).” Stop and play the tape to make sure it is operating and the voice is loud and clear.
5. Let the Interviewee Talk:
Let the person talk. Remember that the interviewee should do most of the talking. It is extremely important that you show interest in what the person is saying. Your body language needs to show interest. You can do this with eye contact and nodding your head. Do not be afraid of occasional silences. Give the interviewee think time. Do not fill the silences with another question. Keep a moderate pace and allow interviewees to completely finish answering a question before asking another. Also, make sure you ask follow up questions. Use your outline of questions, but remain flexible. Always remain unbiased during the interview.
6. Get Consent:
Either at the start or at the end of the interview, go over the “Interview Consent Form”. Getting this form signed by the interviewee is critical because it gives you the right to use the interview in your project. The consent form also informs the interviewee about how you will be using the interview.
7. Word/Name list:
Consider asking the interviewee to help you with spelling keywords, locations, and names. Also inquire about any additional materials such as photographs and diaries that might help you in your History Day reseal.
Survey -are considered the most widely used method for collecting data in descriptive research or in most areas of social inquiry; from politics to sociology, from education to linguistics.
Types of Survey
Public opinion survey- this is the survey of finding out the attitudes, beliefs and opinion of a large number of people.
Social Survey- this is a cooperative undertaking that applies research techniques to the study and analysis of a current social phenomenon, situation or population within definite geographical limits.
School Survey- are conducted to investigate some particular phase or problem of education.
Community Survey- the demarcation line separating social from community survey is very thin.
Educational Survey- this survey is composed of scientific collection and examination of data concerned about educational problems, systematically presented and constructively interpreted for improvement of the instruction.
Job analysis- this type of survey is important for those people who are concerned with building curriculum and teaching young people in preparation for vocation.
Trigonometry
Law of sines
- is a statement about any triangle in a plane. Where the sides of the triangle are a, b and c and the angles opposite those sides are A, B and C, then the law of sines states equality of the first three quantities below:
- underbrace{\frac{a}{\sin A} = \frac{b}{\sin B} = \frac{c}{\sin C}}_\text{Law of sines} = 2R" src="http://upload.wikimedia.org/math/e/3/3/e33da311cee422f675ff12a7110a7709.png">
where R is the radius of the triangle's circumcircle. The law of sines is also sometimes stated as
- frac{\sin A}{a} = \frac{\sin B}{b} = \frac{\sin C}{c}. " src="http://upload.wikimedia.org/math/e/4/5/e457ba26b7d028cc5fd455b5be75cde5.png">
This law is useful when computing the remaining sides of a triangle if two angles and a side are known, a common problem in the technique of triangulation. It can also be used when two sides and one of the non-enclosed angles are known; in this case, the formula may give two possible values for the enclosed angle. When this happens, often only one result will cause all angles to be less than 180°; in other cases, there are two valid solutions to the triangle (see the ambiguous case section of this article for further information).
It can be shown that
- frac{abc} {2S} & {} = \frac{abc} {2\sqrt{s(s-a)(s-b)(s-c)}} \\ & {} = \frac {2abc} {\sqrt{(a^2+b^2+c^2)^2-2(a^4+b^4+c^4) }}, \end{align}" src="http://upload.wikimedia.org/math/0/8/9/0890b2eb7f73929d9048a5d95ee68874.png">
where S is the area of the triangle and s is the semiperimeter
- frac{a+b+c} {2}." src="http://upload.wikimedia.org/math/e/c/5/ec50234ccbbd096784304c4048bc55a7.png">
- law of cosines
- -is a statement about a general triangle which relates the lengths of its sides to the cosine of one of its angles. Using notation as in Fig. 1, the law of cosines states that

or, equivalently:
- bc\cos(\alpha), \," src="http://upload.wikimedia.org/math/f/5/e/f5e7ac224e943897617704077a13dd7b.png">
- frac{a^2 + b^2 - c^2}{2ab}, \," src="http://upload.wikimedia.org/math/7/1/2/712fe5ff86ed1b495704f73a3552d96d.png">
- frac{a^2 + c^2 - b^2}{2ca}, \," src="http://upload.wikimedia.org/math/6/0/2/602ef2e6bf70ccad4b941768609c9f91.png">
- frac{b^2 + c^2 - a^2}{2bc}. \," src="http://upload.wikimedia.org/math/d/6/e/d6ef13cdf8bdae84c3429222fc14aede.png">
Note that c is the side opposite of angle γ, and that a and b are the two sides enclosing γ. All three of the identities above say the same thing; they are listed separately only because in solving triangles with three given sides one may apply the identity three times with the roles of the three sides permuted.
The law of cosines generalizes the Pythagorean theorem, which holds only for right triangles: if the angle γ is a right angle (of measure 90° or π/2 radians), then cos(γ) = 0, and thus the law of cosines reduces to
which is the Pythagorean theorem.
The law of cosines is useful for computing the third side of a triangle when two sides and their enclosed angle are known, and in computing the angles of a triangle if all three sides are know.
Data structure
- a mixture of a natural language and high level programming concept that described the main ideas behind a genetic implementation of a data structure or algorithm
- it is more structured than usual prose but less formal than a programming language.
stacks- a last in first out (LFO) dta structure" Inside the stacks"
Push- inserting or placing an item inside the stack.
Pop- removes an item from the stacks
- remove the top element of the stacks.
Top- examines the top element of the stacks.
Empty- return true if and only if the stacks contains nothing. Null (value is 0).
Evaluation of expressions
-MDAS RULE
- if the outside is greater push (outside)
-if outside is lower/or equal -Pop(top)
- Push(Outside)
1. Infix Notation
- operand operator operand
2. Post Fix Notation
- operand operand operator
3. Prefix Notation
- operator operand operand
Queues- is a sequential organization of data.
Head(Front)- examine the front/head item of the queue.
De queue- remove the front item from the queue.
Empty- return true if and only if the queue contains nothing.
2 Kinds of Queue.
Simple and Priority- organized items in a line where the first item is at the back of the line.
Priority queue- is similar to a simple queue in the items are organized in a line and processed sequentially.
Link List-is one of the fundamental data structures, and can be used to implement other data structures. It consists of a sequence of nodes, each containing arbitrary data fields and one or two references ("links") pointing to the next and/or previous nodes. The principal benefit of a linked list over a conventional array is that the order of the linked items may be different from the order that the data items are stored in memory or on disk, allowing the list of items to be traversed in a different order. A linked list is a self-referential data type because it contains a pointer or link to another datum of the same type. Linked lists permit insertion and removal of nodes at any point in the list in constant time, but do not allow random access.
Types of linked List
Linearly linked list
The simplest kind of linked list is a singly-linked list (or slist for short), which contains a node having two fields, one is information field and another is link field. This link points to the next node in the list, and last node points to a null value
![]()
A singly-linked list containing two values: the value of the current node and a link to the next node
A singly linked list's node is divided into two parts. The first part holds or points to information about the node, and second part holds the address of next node. A singly linked list travels one way.
Doubly-linked list
A more sophisticated kind of linked list is a doubly-linked list or two-way linked list. Each node has two links: one points to the previous node, or points to a null value or empty list if it is the first node; and one points to the next, or points to a null value or empty list if it is the final node.
A doubly-linked list containing three integer values: the value, the link forward to the next node, and the link backward to the previous node
In some very low level languages, XOR-linking offers a way to implement doubly-linked lists using a single word for both links, although the use of this technique is usually discouraged.
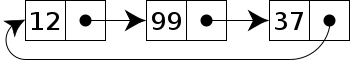
Circularly-linked list
In a circularly-linked list, the first and final nodes are linked together. This can be done for both singly and doubly linked lists. To traverse a circular linked list, you begin at any node and follow the list in either direction until you return to the original node. Viewed another way, circularly-linked lists can be seen as having no beginning or end. This type of list is most useful for managing buffers for data ingest, and in cases where you have one object in a list and wish to iterate through all other objects in the list in no particular order.
The pointer pointing to the whole list may be called the access pointer.
2. Every other node has exactly one parent.
Sibling- 2 nodes with the same parent
Internal node- node with at least 1 child.
External node- node without children.
Ancestor-parent , grandparent, grand- grandparent.
Depth- number of ancestor.
Descendant- Child granchild, grand- grandchild.
Binary Tree Traversal
Traversal- is the process of visitng every node once.
Technique:
Pre order- the root is visited before the sub tree traversal.
Rules: Root, Left Right
In order- the root is visited in between left and right sub tree traversal.
Rules: Left, Root, Right
Post order- the root visited after the sub tree traversal.
Rules:Left, right root
Sorting -is an algorithm that puts elements of a list in a certain order. The most-used orders are numerical order and lexicographical order. Efficient sorting is important to optimizing the use of other algorithms (such as search and merge algorithms) that require sorted lists to work correctly; it is also often useful for canonicalizing data and for producing human-readable output. More formally, the output must satisfy two conditions:
- The output is in nondecreasing order (each element is no smaller than the previous element according to the desired total order);
- The output is a permutation, or reordering, of the input.
Bubble sort-is a straightforward and simplistic method of sorting data that is used in computer science education. The algorithm starts at the beginning of the data set. It compares the first two elements, and if the first is greater than the second, it swaps them.
Insertion sort - is a simple sorting algorithm that is relatively efficient for small lists and mostly-sorted lists, and often is used as part of more sophisticated algorithms. It works by taking elements from the list one by one and inserting them in their correct position into a new sorted list
Shell Sort- It improves upon bubble sort and insertion sort by moving out of order elements more than one position at a time. One implementation can be described as arranging the data sequence in a two-dimensional array and then sorting the columns of the array using insertion sort.
Merge sort- takes advantage of the ease of merging already sorted lists into a new sorted list. It starts by comparing every two elements (i.e., 1 with 2, then 3 with 4...) and swapping them if the first should come after the second. It then merges each of the resulting lists of two into lists of four, then merges those lists of four, and so on; until at last two lists are merged into the final sorted list. Of the algorithms described here, this is the first that scales well to very large lists, because its worst-case running time is O(n log n).
Heapsort- is a much more efficient version of selection sort. It also works by determining the largest (or smallest) element of the list, placing that at the end (or beginning) of the list, then continuing with the rest of the list, but accomplishes this task efficiently by using a data structure called a heap, a special type of binary tree.
About mY Self...............................................
Im rhea ringor, 18 years of age student of Agoo Computer College Philippines. I came from Sablayan Occidental Mindoro, but this time i leave in Sta. ana Agoo, la Union in the house of my uncle. This time im studying through the help of my uncle because my parents can't afford to support my studying. So my parents and my uncle decide that i continue my studies through his support.



No comments:
Post a Comment